Carnot Cycle & Engines!

Here we are shown a PV graph and asked to find the W.

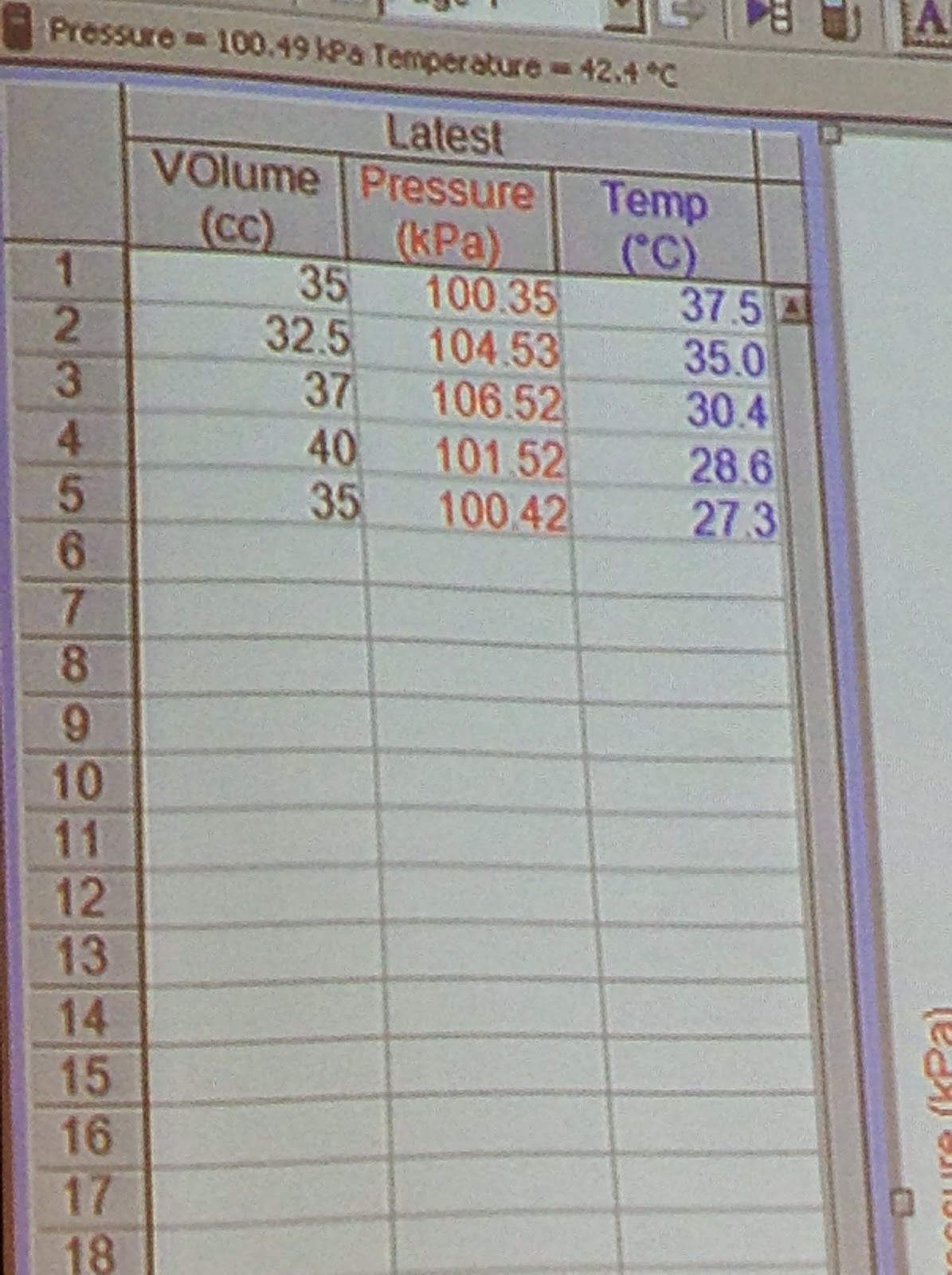Close up shot of Values

Unfortunately we were not able to solve for the W in class, however we did learn that work is the area in the shape. So we should have approached it by seperating the Graph into Trapezoids i would use 6 one from 32-35 the other from 35-37 and lastly 37-40, then i would subtract the bottom trapezoids giving us 6 in total.

Here we can use our definitions of internal energy work and heat, to solve for the constant Cp=5/2R.
We can also do the same for Cv by letting work = 0 and Q=nCvdelT giving us Cv=3/2R
Note: Cp-Cv=R

Here we have an adiabatic Expansion with small changes in pressure and volume. we use the chain rule to solve for ndelT, and then we substitute our Cp-Cv for R.using our Definition for adiabatic expansion we let Q=0 then set our definitions of Energy Internal to write a new equation. From here we set our two new equations equal to each other and take the the integral.

Now we can show relationships between P T and V. by using our ideal gas law PV=nRT.
Note: gamma = Cp/Cv

Using our new Formula for Pressure initial we can now solve for W=Spdv.

Here we solve for DelE Q and W by using our new formulas we just derived for the adiabatic expansion part of the carnot cycle. There were a few givens like Q=0 for adibatic and for Isothermal (U=3/2nKbdelT) Internal Energy is 0. From there we just used Wadiabatic to solve for W and E internal for two of the processes. The Isothermal Processes we used Eint = nCvdelT and then solved for W since Q=0. To find the efficiency of the system  we can W/Qh.